Authors:
(1) Cameron Parker, Cyclotron Institute, Texas A&M University and Department of Physics and Astronomy, Texas A&M University (E-mail: [email protected]);
(2) JETSCAPE Collaboration.
Table of Links
3. Medium Effects
In this section we use a simplified event pipeline. Since we are not interested in the entire event, only how the medium affects hadronization, we only examine a single jet in a quark gluon plasma medium. This is done by firing a single parton in the 𝑥-direction through a medium with a set length and temperatured (a "brick"), showering the parton with MATTER and LBT, and then hadronizing the shower with Hybrid Hadronization. Flow is emulated by adding a set velocity to the thermal partons at the hadronization stage. In the following, longitudinal is defined as in the direction of the jet (𝑥) and transverse is perpendicular to the jet.
We first examine the effects of the presence of thermal partons during hadronization. We plot the proton-to-pion ratio as a function of hadron momentum 𝑝𝑥 for a variety of different brick lengths from 0 (vacuum) to 8 fm. A large proton-to-pion ratio is known as a signature of quark
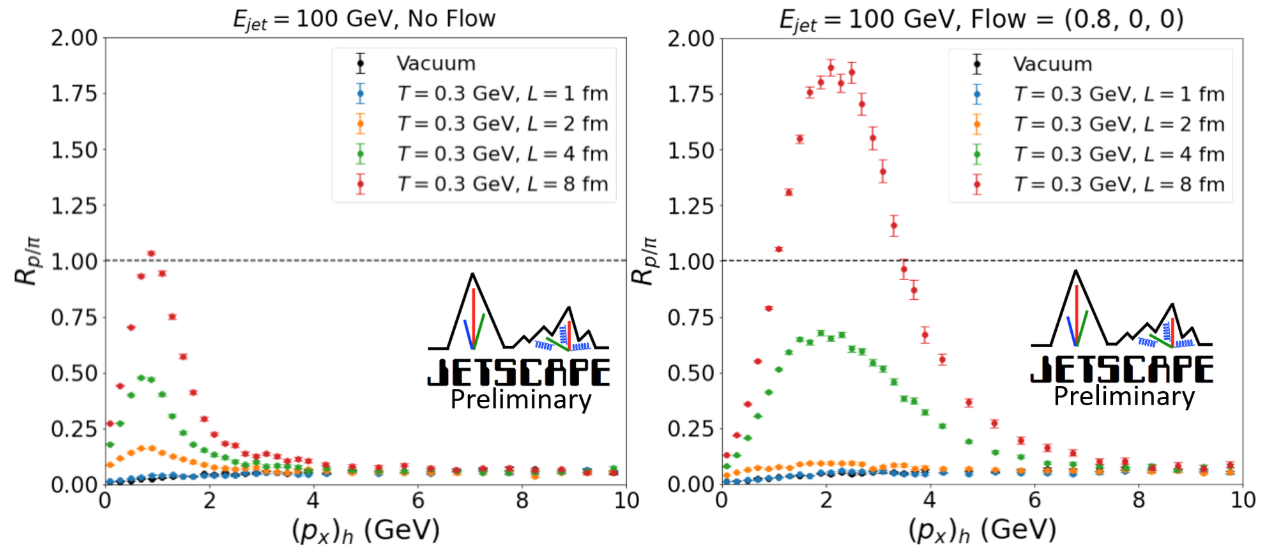
recombination in hadronization. As shown in the left panel of Fig. 3, the proton-to-pion ratio around 1 GeV indeed increases in magnitude as the brick increases in size. This is consistent with the idea that recombination with thermal partons increases in a larger medium.
When a homogenous longitudinal flow in jet direction is added, the peaks in the proton-to-pion ratios grow and are shifted to 2-2.5 GeV. This can be understood by flowing thermal partons adding more momentum to the hadrons they recombine into. Similar effects can be seen in the Λ-to-𝐾 ratio, not shown here.
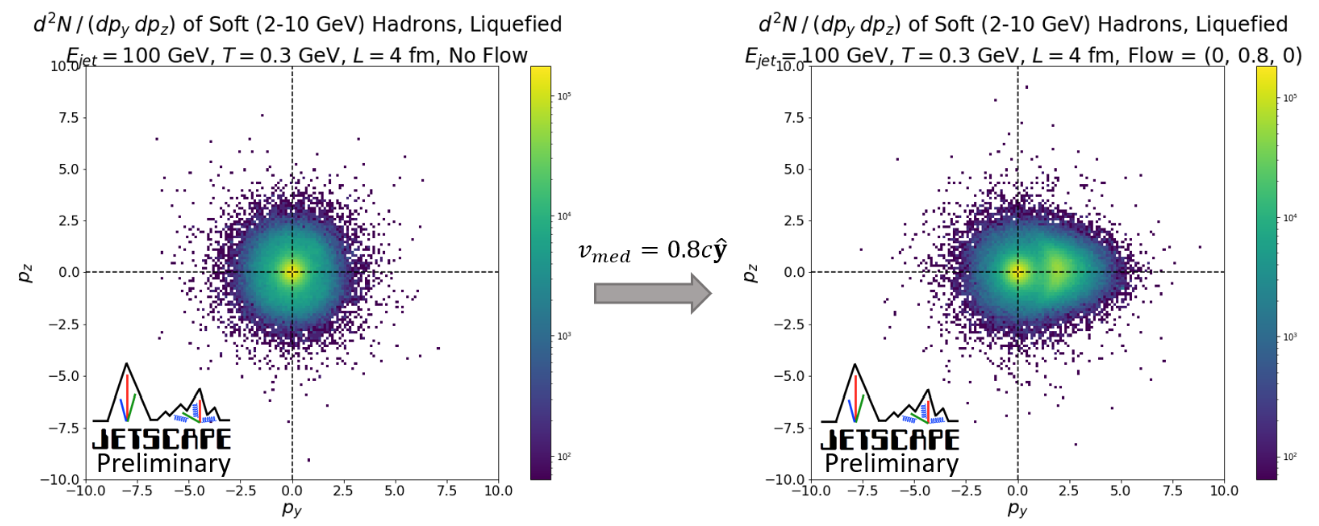
We then examine the effects of transverse flow of brick partons. We scatter plot the components of the momentum perpendicular to the jet (𝑝𝑦 and 𝑝𝑧) for each hadron to showcase the effects of the flow. Soft hadrons, defined as 2 GeV < 𝑝𝑥 < 10 GeV, demonstrate a significant deflection in the direction of the flow as shown in Fig. 4. On the other hand, leading hadrons demonstrate no noticeable deflection. This is consistent with expectation. Leading partons are distant from thermal partons in phase space, and therefore have a negligible chance to recombine with them.
4. Conclusion

Our study of medium effects in jet hadronization is similarly promising. Using a brick with flow we reproduce all the expected effects, including baryon enhancement increasing with medium size, a shift of the baryon/meson peak in momentum with longitudinal flow, and sideways deflection of soft hadrons with transverse flow. We intend to progress on to heavy flavor jets and simulations of jets in full 𝐴 + 𝐴 collisions.
This work was supported by the U.S. National Science Foundation under awards 1812431 and 2111568, and under award 2004571 through a subcontract with Wayne State University.
References
[1] A. Kumar, et al. Phys. Rev. C 102, no.5, 054906 (2020), doi:10.1103/PhysRevC.102.054906, [arXiv:1910.05481 [nucl-th]].
[2] K. C. Han, R. J. Fries and C. M. Ko, Phys. Rev. C 93, no.4, 045207 (2016), doi:10.1103/PhysRevC.93.045207, [arXiv:1601.00708 [nucl-th]].
[3] R. J. Fries and M. Kordell, PoS HardProbes2018, 046 (2019) doi:10.22323/1.345.0046 [arXiv:1901.08157 [nucl-th]].
[4] R. J. Fries, B. Müller, C. Nonaka and S. A. Bass, Phys. Rev. Lett. 90, 202303 (2003), doi:10.1103/PhysRevLett.90.202303, [arXiv:nucl-th/0301087 [nucl-th]].
[5] R. J. Fries, B. Müller, C. Nonaka and S. A. Bass, Phys. Rev. C 68, 044902 (2003), doi:10.1103/PhysRevC.68.044902, [arXiv:nucl-th/0306027 [nucl-th]].
[6] B. Andersson, G. Gustafson, G. Ingelman and T. Sjostrand, Phys. Rept. 97, 31-145 (1983), doi:10.1016/0370-1573(83)90080-7.
[7] C. Bierlich, S. Chakraborty, N. Desai, L. Gellersen, I. Helenius, P. Ilten, L. Lönnblad, S. Mrenna, S. Prestel and C. T. Preuss, et al., doi:10.21468/SciPostPhysCodeb.8, [arXiv:2203.11601 [hep-ph]].
[8] A. Majumder, Phys. Rev. C 88, 014909 (2013), doi:10.1103/PhysRevC.88.014909, [arXiv:1301.5323 [nucl-th]].
[9] S. Cao and A. Majumder , Phys. Rev. C 101, no.2, 024903 (2020), doi:10.1103/PhysRevC.101.024903, [arXiv:1712.10055 [nucl-th]].
[10] V. Khachatryan, et al., Phys. Rev. C 96, no.1, 015202 (2017), doi:10.1103/PhysRevC.96.015202, [arXiv:1609.05383 [nucl-ex]].
[11] The CMS Collaboration, S. Chatrchyan, V. Khachatryan, et al., Eur. Phys. J. C 72, 1945 (2012), doi:10.1140/epjc/s10052-012-1945-x, [arXiv:1202.2554 [nucl-ex]].
[12] PHENIX Collaboration, A. Adare, et al., Phys. Rev. D 76, no.5, 051106 (2007), doi:10.1103/physrevd.76.051106, [arXiv:0704.3599 [hep-ex]].
This paper is available on arxiv under CC BY 4.0 DEED license.

